What is Ohm's Law?

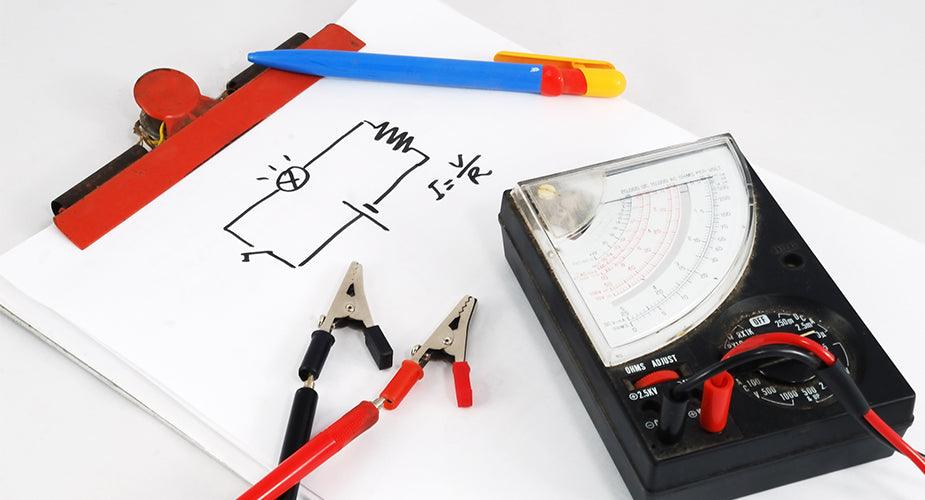
When you talk about electricity, one of the first concepts you'll encounter is Ohm's Law. But what is Ohm's Law, and why is it so important? Simply put, Ohm's Law is a fundamental principle that helps you understand how electrical circuits work. It explains the relationship between voltage, current, and resistance, which are the three basic building blocks of electricity. Understanding Ohm's Law is important because it allows you to predict how electrical systems will behave, making it easier to design and troubleshoot circuits. Whether you're an aspiring electrician, a student, or just curious about how things work, learning this concept will help your journey through the fascinating realm of electricity.

Explanation of Ohm's Law

So, what is Ohm's Law? Ohm's Law is a simple formula that explains the relationship between current, voltage, and resistance in a conductor. It states that the current flowing through a conductor is directly related to the voltage across it and inversely related to the resistance.

V=I×RV = I \times RV=I×R

• Voltage (V) is the electrical potential difference between two points in a circuit. Think of it as the force that pushes electric charges through a conductor.
• Current (I) is the flow of electric charge through a conductor, measured in amperes (A). It's like the amount of water flowing through a pipe.
• Resistance (R) is the opposition to the flow of current, measured in ohms (Ω). It's similar to the friction that slows down the flow of water in a pipe.

Understanding this relationship is key to figuring out how much current will flow for a given voltage and resistance. For instance, if you know the voltage and the resistance in a circuit, you can use Ohm's Law to calculate the current. This makes it an invaluable tool for anyone working with electrical systems, from engineers to hobbyists.

Practical applications

Now that you know what Ohm's Law is, let's explore how you can use it in real-world situations. Ohm's Law is a cornerstone in electrical engineering and is applied in numerous practical scenarios.

For instance, imagine you're designing a simple circuit with a battery and a resistor. If you know the battery provides 9 volts (V) and the resistor has a resistance of 3 ohms (Ω), you can use Ohm's Law to determine the current flowing through the circuit. Using the formula V=I×RV = I \times RV=I×R, you can rearrange it to I=VRI = \frac{V}{R}I=RV​. Plugging in the numbers, you get I=9V3ΩI = \frac{9V}{3Ω}I=3Ω9V​, which results in a current of 3 amperes (A).

Here's another example: Suppose you're troubleshooting a circuit and need to find the resistance. You measure the voltage at 12 volts and the current at 2 amperes. Rearranging Ohm's Law to solve for resistance, you get R=VIR = \frac{V}{I}R=IV​. Substituting the known values, R=12V2AR = \frac{12V}{2A}R=2A12V​, you find the resistance is 6 ohms.

Ohm's Law is also highly relevant in modern applications like solar generators. Solar panels generate a certain amount of voltage depending on the sunlight they receive. Understanding this voltage, along with the current and resistance in the system, helps you design and optimize solar generators. For example, if you need to power a device, you can calculate the required voltage and current and select appropriate solar panels and components accordingly. Additionally, knowing what is Ohm's Law helps troubleshoot issues within the solar generator system, ensuring safe and efficient operation by maintaining current and voltage levels within safe limits.

These simple calculations show how Ohm's Law helps you design circuits and solve problems. It's essential in tasks like determining the correct resistor to use, ensuring components won't overheat, and making sure your circuits operate safely and efficiently.

Extension and limitations

While Ohm's Law is a basic principle in understanding electrical circuits, it's important to recognize that it has its limitations and doesn't apply in all situations. There are specific conditions and factors that might not hold Ohm's Law true.

Circumstances and Conditions

1. Non-Ohmic Materials: Ohm's Law applies to ohmic materials, where the relationship between voltage, current, and resistance is linear. However, some materials, known as non-ohmic materials, do not follow this linear relationship. Examples include diodes and transistors, which have varying resistance depending on the voltage and current.
2. High-Frequency AC Circuits: In alternating current (AC) circuits with high frequencies, the effects of capacitance and inductance become significant. These elements introduce reactance, a type of resistance that varies with frequency, complicating the simple linear relationship described by Ohm's Law.
3. Temperature Variations: Materials can change their resistance as the temperature changes. For example, when the temperature of a conductor goes up, its resistance also increases. This means that the connection between voltage and current can change when the temperature changes. This can cause the behavior of the conductor to deviate from what is Ohm's Law under varying thermal conditions.
4. Strong Electric Fields: In very strong electric fields, the behavior of materials can change. For example, in semiconductors or at very high voltages, the resistance might not remain constant, and Ohm's Law might not accurately describe the relationship between voltage and current.

Other Factors Affecting Electrical Systems

1. Capacitance and Inductance: In more complex circuits, capacitance (stored energy in electric fields) and inductance (stored energy in magnetic fields) play significant roles. These factors introduce phase differences between voltage and current, requiring more advanced principles beyond Ohm's Law to fully describe circuit behavior.
2. Power Considerations: Ohm's Law primarily deals with voltage, current, and resistance, but in practical applications, power (the rate at which energy is used or transferred) is also crucial. The power in a circuit is given by P=V×IP = V \times IP=V×I, and understanding power dynamics often involves additional principles alongside Ohm's Law.

Despite these limitations, Ohm's Law remains a valuable and widely applicable tool for understanding and working with electrical circuits. It provides a foundational framework that, when combined with knowledge of its limitations and extensions, allows for a comprehensive understanding of electrical systems.

Conclusion

Ohm's Law is a key principle that explains how voltage, current, and resistance interact in electrical circuits. It's important for anyone working with electricity, from designing and troubleshooting circuits to optimizing systems like solar generators. While it doesn't apply in every situation, this will help you understand and predict how electrical systems will behave. By mastering what is Ohm's Law, you gain valuable insights into the basics of electricity, making it easier to innovate and solve problems in electronics and electrical engineering. Whether you're learning, working, or just curious, Ohm's Law is a fundamental tool in your electrical toolkit.